function thomas_attractor

deeptime.data.thomas_attractor(b: float = 0.15, h: float = 0.001, n_steps: int = 1000)

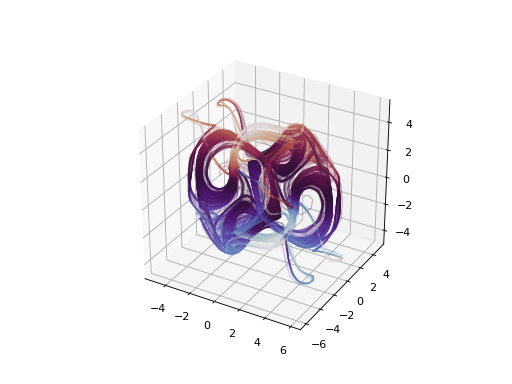
The Thomas attractor. [1]

It lives in three dimensions and is generated by the dynamical system

x˙=sin⁡(y)−bxy˙=sin⁡(z)−byz˙=sin⁡(x)−bz\begin{aligned} \dot{x} &= \sin(y) - bx\\ \dot{y} &= \sin(z) - by \\ \dot{z} &= \sin(x) - bz \end{aligned}

with a free parameter bb, determining the dissipativity of the system. A value of b>1b > 1 yields a single stable equilibrium, at values of b≤1b \leq 1 the system has at least two attractive fixed points.

(Source code, png, hires.png, pdf)

../../_images/plot_thomas_attractor.png
Parameters:

  • b (float) – Dissipativity.

  • h (float) – Step size for the integrator.

  • n_steps (int) – Number of integration steps per evaluation / recording of the state.

Returns:

system – The system.

Return type:

TimeIndependentSystem

References